V ( ,t) ,t) | = 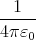 ∫ ∫
 d3 d3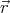 ′ ′ | ||
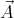 ( (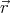 ,t) ,t) | =  ∫ ∫
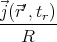 d3 d3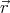 ′, ′, |
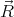 =
= 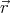 -
-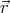 ′ and tr = t - R∕c, satisfy the Lorenz gauge condition:
′ and tr = t - R∕c, satisfy the Lorenz gauge condition:
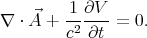
(Note: d3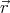 ′ denotes volume integration with respect to the vector
′ denotes volume integration with respect to the vector
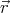 ′.)
′.)
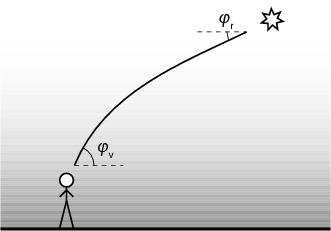
- Show that in a static electric field a point charge cannot have a stable equilibrium point in vacuum!
- Show that in a static magnetic field a tiny magnet cannot have a
stable equilibrium point in vacuum. First solve the problem in the
simpler case when the spatial orientation of the magnet is fixed
(i.e. its magnetic moment
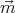 = const.. Then solve the problem in
the general case when the magnet can rotate freely.
= const.. Then solve the problem in
the general case when the magnet can rotate freely.
|
|
An electromagnetic wave is travelling through a plasma, which contains n electrons per unit volume. The electrons are brought into motion by the electric field, but the ions, which have a much lower specific charge, can be safely considered stationary.
Calculate the relative permittivity εr of the plasma as a function of the angular frequency ω of the electromagnetic wave.
Hint: The Taylor expansion of the logarithm function will prove helpful.
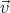 (
(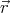 ))! (See
fig. 2.)
))! (See
fig. 2.)
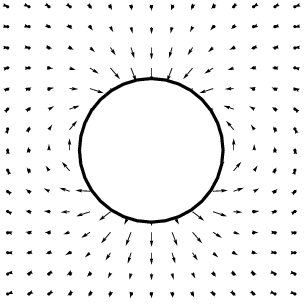
Assume that water is incompressible (its density is constant), and that the
flow is irrotational (∇×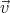 = 0). These assumptions are rarely correct for real
water, however, if the ball is falling neither “too fast” (there is no
turbulence), nor “too slow” (the viscous forces are negligible), then we can
get an approximation about how the water is flowing around the
ball.
= 0). These assumptions are rarely correct for real
water, however, if the ball is falling neither “too fast” (there is no
turbulence), nor “too slow” (the viscous forces are negligible), then we can
get an approximation about how the water is flowing around the
ball.
Hint: There is an electrostatic analogue for the equations!