3rd of September, 2008

1. $\overrightarrow{a}\perp \overrightarrow{b}$, so $\overrightarrow{a}\cdot \overrightarrow{b}=0$ and $\overrightarrow{v}\cdot \overrightarrow{a}=\alpha$. Multiplying the first equation by $\overrightarrow{a}$ from the left (using the cross product), we get $\overrightarrow{a}\times (\overrightarrow{v}\times \overrightarrow{a})=\overrightarrow{a}\times \overrightarrow{b}\iff a^2\overrightarrow{v}-\overrightarrow{a}(\overrightarrow{v}\cdot \overrightarrow{a})=\overrightarrow{a}\times \overrightarrow{b}$. Using that $\overrightarrow{v}\cdot \overrightarrow{a}=\alpha$,
   $$\overrightarrow{v}=\frac{\overrightarrow{a}\times \overrightarrow{b}+\alpha \overrightarrow{a}}{a^2}$$

2. 1. $$\nabla \cdot \left(\right(\overrightarrow{b}\cdot \overrightarrow{r}\left)\overrightarrow{a}\right)=\overrightarrow{b}\cdot \overrightarrow{a}\nabla \times \left(\right(\overrightarrow{b}\cdot \overrightarrow{r}\left)\overrightarrow{a}\right)=\overrightarrow{b}\times \overrightarrow{a}$$

   2. $$\nabla \cdot \overrightarrow{r}=3\nabla \times \overrightarrow{r}=0$$

   3. $$\nabla \cdot \left(r\overrightarrow{r}\right)=4r\nabla \times \left(r\overrightarrow{r}\right)=0$$

      (Note: The curl of any radially symmetric vector field is 0.)

   4. $$\nabla \cdot (\overrightarrow{a}\times (\overrightarrow{b}\times \overrightarrow{r}\left)\right)=-2\overrightarrow{a}\cdot \overrightarrow{b}\nabla \times (\overrightarrow{a}\times (\overrightarrow{b}\times \overrightarrow{r}\left)\right)=\overrightarrow{a}\times \overrightarrow{b}$$

   5. $$\nabla \cdot \left(\right(\overrightarrow{a}\cdot \overrightarrow{r}\left)\right(\overrightarrow{b}\times \overrightarrow{r}\left)\right)=(\overrightarrow{a}\times \overrightarrow{b})\cdot \overrightarrow{r}\nabla \times \left(\right(\overrightarrow{a}\cdot \overrightarrow{r}\left)\right(\overrightarrow{b}\times \overrightarrow{r}\left)\right)=3\overrightarrow{b}(\overrightarrow{a}\cdot \overrightarrow{r})-\overrightarrow{r}(\overrightarrow{a}\cdot \overrightarrow{b})$$

   6. $$\nabla \cdot (\overrightarrow{r}\times (\overrightarrow{a}\times \overrightarrow{r}\left)\right)=-2\overrightarrow{a}\cdot \overrightarrow{r}\nabla \cdot (\overrightarrow{r}\times (\overrightarrow{a}\times \overrightarrow{r}\left)\right)=3\overrightarrow{r}\times \overrightarrow{a}$$

   The curl of an electrostatic field is 0, so if $\overrightarrow{a}$ and $\overrightarrow{b}$ are not parallel, then only the fields in points (b) and (c) may be electrostatic fields.

3. 1. The charge density is

      $$\begin{array}{cc}\begin{array}{rl}\rho & ={\epsilon }_0\nabla \cdot \overrightarrow{E}\\ & ={\epsilon }_0\nabla \cdot \left(A\frac{e^{-br}}{r^3}\overrightarrow{r}\right)\\ & ={\epsilon }_{0}A(\nabla e^{-br})\frac{\overrightarrow{r}}{r^3}+{\epsilon }_{0}A{e}^{-br}\left(\nabla \cdot \frac{\overrightarrow{r}}{r^3}\right)\end{array}& \end{array}$$

      Let us calculate the two terms of this sum separately:

      $$\nabla e^{-br}=-b{e}^{-br}\widehat{r},$$

      where $\widehat{r}=\overrightarrow{r}∕r$, and

      $$\nabla \cdot \frac{\overrightarrow{r}}{r^3}=\nabla \cdot \frac{\widehat{r}}{r^2}=4\pi \delta \left(\overrightarrow{r}\right).$$

      Thus the complete charge density is

      $$\rho \left(\overrightarrow{r}\right)=4\pi {\epsilon }_{0}A\delta \left(\overrightarrow{r}\right)-{\epsilon }_{0}Ab\frac{e^{-br}}{r^2}.$$

   2. The total charge enclosed by a spherical surface $S$ of radius $r$ centred around the origin is
      $$Q\left(r\right)={\epsilon }_0{\oint }_S\overrightarrow{E}\cdot \overrightarrow{S}={\epsilon }_{0}A{e}^{-br}\frac{1}{r^2}4\pi r^2=4\pi {\epsilon }_{0}A{e}^{-br}.$$

      Thus as $r\to \infty$, the total charge enclosed by the surface goes to 0.

4. The work needed to add another layer of charge $dq$ to a sphere of radius $r$ and total charge $q$ is
   $$dW=\frac{1}{4\pi {\epsilon }_0}\frac{qdq}{r}$$

   The sphere has a homogeneous charge distribution, so $q=\frac{4\pi }{3}{r}^3\rho$ and $dq=4\pi \rho r^{2}dr$.

   Thus the energy needed to assemble a charged sphere of radius $R$ and charge $Q$ is

   $${\int \; <!--nolimits-->}_0^{R}dW=\frac{4\pi {\rho }^2{R}_0^5}{15{\epsilon }_0}=\frac{3}{20\pi {\epsilon }_0}\frac{Q^2}{R}.$$

   ---

   Figure 1: The potential and electric field as a function of the distance from the centre of the sphere.

   ---

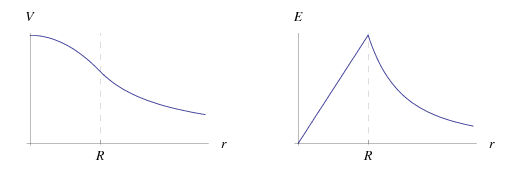
   The electric field is

   $$\overrightarrow{E}\left(r\right)=\frac{Q}{4\pi {\epsilon }_0}\times \left\{\begin{array}{cc}r∕R^3\hfill & \text{ if }r\le R\hfill \\ 1∕r^2\hfill & \text{ if }r\ge R\hfill \end{array}\right.,$$

   and the potential is

   $$V\left(r\right)=\frac{Q}{4\pi {\epsilon }_0}\times \left\{\begin{array}{cc}(3R^2-r^2)∕\left(2R^3\right)\hfill & \text{ if }r\le R\hfill \\ 1∕r\hfill & \text{ if }r\ge R\hfill \end{array}\right..$$

5. Let us find the gravitational potential energy $W\left(x\right)$ of the two half-spheres as a function of their separation $x$. The force keeping them together is simply $F=dW∕dx$.

   Creating a gap by separating the halves is equivalent to removing (“mining out”) all the matter from the gap, and distributing it on the surface (i.e. it causes the same change $\Delta W$ in energy). Provided that the gap between the halves is very narrow compared to the planet’s radius ($x\ll R$), the change in the gravitational field is negligible.

   Using Gauß’s law, it is easily shown that the gravitational potential below the surface of the spherical planet is

   $$V\left(r\right)=\gamma \frac{M}{2R^3}{r}^2+\mathrm{\text{ const.}},$$

   where $\gamma$ is the gravitational constant, $M$ is the mass of the planet, and $r$ is the distance from the centre.

   When moving a piece of mass $dm$ up to the surface, the increase in energy is $\left(V\right(R)-V(r\left)\right)dm$. To get the total change in energy, we must integrate over the volume of the gap:

   $$\begin{array}{cc}\begin{array}{rl}\Delta W\left(x\right)& ={\int \; <!--nolimits-->}_{\mathrm{\text{ (gap)}}}\left(V\right(R)-V(r\left)\right)dm\\ & =x\frac{\gamma M}{2R^3}\frac{M}{\frac{4}{3}\pi R^3}{\int \; <!--nolimits-->}_0^R(R^2-r^2)2\pi rdr\\ & =x\frac{3\gamma M^2}{16R^2}.\end{array}& \end{array}$$

   (1)

   The force keeping the halves together is

   $$F=\frac{dW\left(x\right)}{dx}=\frac{dW\left(x\right)}{dx}-\underset{0}{\underbrace{\frac{dW\left(0\right)}{dx}}}=\frac{d\Delta W}{dx}=\frac{3\gamma M^2}{16R^2}.$$

   (I.e. the same as the weight of an object of mass $\frac{3}{16}M$ on the surface of the planetoid.)

Back to the main page